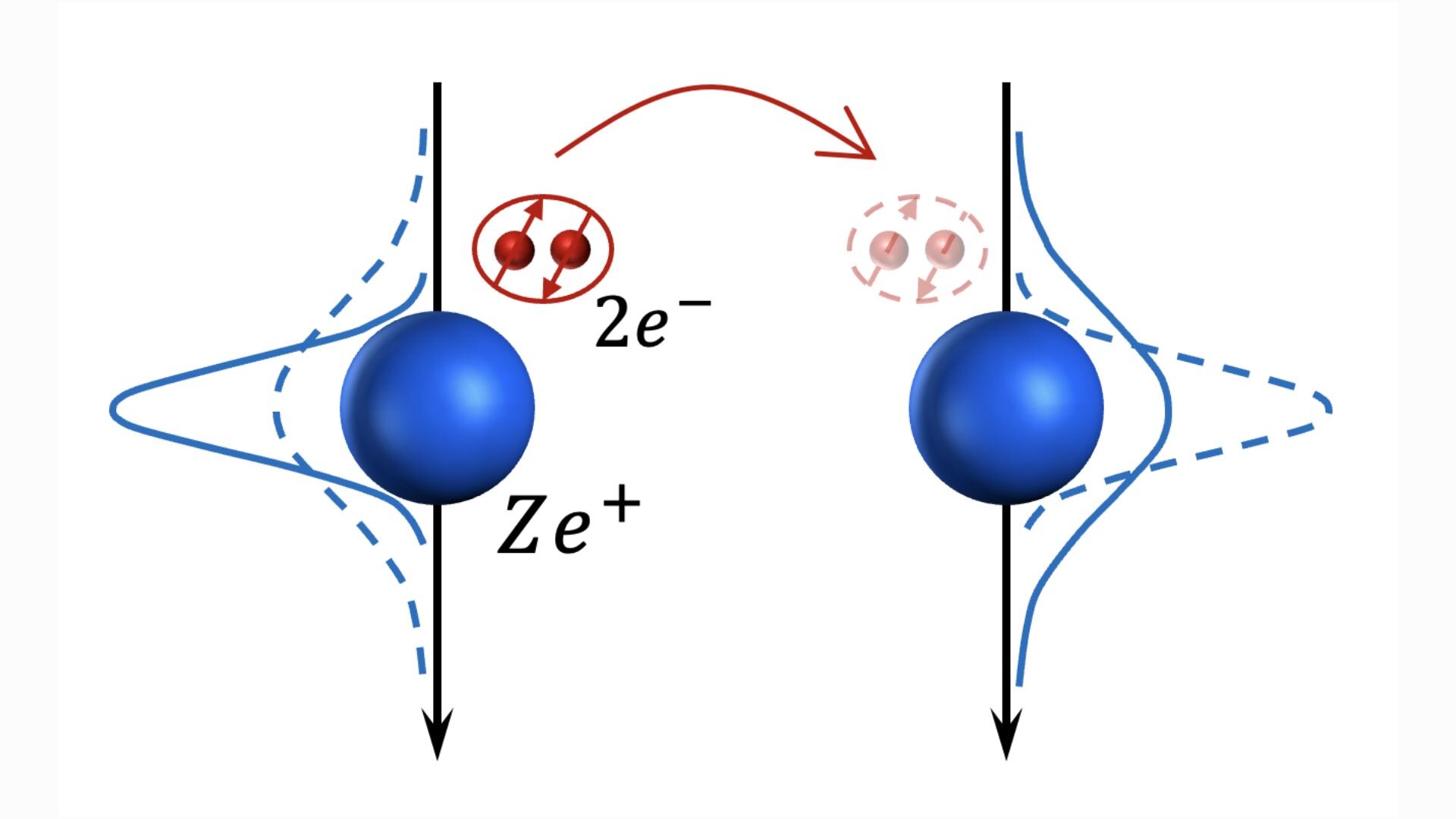
Conceptual image of the formation of quantum bipolarons. The blue spheres represent the positively charged ions in the material’s lattice and the two red dots are the Cooper pairs. Credit: Pavel A. Volkov.
A new study published in Physical Assessment Letters (PRL) investigates the potential of quadratic electron-phonon coupling to enhance superconductivity through the formation of quantum bipolarons.
Electron-phonon coupling is the interaction between electrons and vibrations in a lattice called phonons. This interaction is crucial for superconductivity (resistance-free electrical conduction) in certain materials because it facilitates the formation of Cooper pairs.
Cooper pairs are pairs of electrons that are bonded together through attractive interactions. When these Cooper pairs condense into a coherent state, we get superconducting properties.
Electron-phonon coupling can be categorized based on its dependence on phonon displacement, meaning how much the lattice vibrates. The most commonly considered case is when electron density couples linearly to lattice displacements, creating a lattice distortion surrounding each electron.
The researchers wanted to investigate whether superconductivity can be improved for materials that exhibit quadratic coupling, meaning that the interaction energy is proportional to the square of the phonon displacement.
Phys.org spoke with the study’s co-authors, Zhaoyu Han, a Ph.D. candidate at Stanford University and Dr. Pavel Volkov, Assistant Professor in the Department of Physics, University of Connecticut.
Speaking about his motivation behind pursuing this research, Han said, “It has been one of my dreams to identify and propose new mechanisms that can help achieve superconductivity at high temperatures.”
Dr. Volkov said: “The superconductivity of doped strontium titanate was discovered more than 50 years ago, but its mechanism remains an open question, with conventional mechanisms unlikely. Therefore, I started investigating alternative electron-phonon coupling mechanisms.”
Linear coupling and its challenges for superconductivity
As mentioned earlier, coupling can be categorized as linear or quadratic coupling.
Linear coupling refers to the scenario where the coupling is proportional to the displacement of the phonons. On the other hand, quadratic coupling depends on the square of the phonon displacement.
They can be identified by studying the symmetry of the material, experimental observations and theoretical frameworks. However, their implications for superconductivity seem very different.
Linear coupling, seen in most superconducting materials, is extensively studied due to its prevalence in many materials and has a theoretical framework.
However, conventional superconductors with linear electron-phonon coupling face limitations. These materials have a low critical temperature, which is the temperature below which the material can exhibit superconductivity.
Han explained: “The critical temperatures for these superconductors are usually below 30 Kelvin or -243.15 degrees Celsius. This is partly because the Cooper pair binding energy and kinetic energy are exponentially suppressed in the weak and strong coupling regimes, respectively. .”
In weak coupling, the electron-phonon interactions are weak due to the low binding energy. In strong coupling, the interactions are stronger, leading to a higher effective mass of the Cooper pairs, suppressing the superconductivity.
However, the suppression hinders all attempts to improve the critical temperatures in such materials by merely increasing the coupling strength, encouraging researchers to explore materials with quadratic electron-phonon coupling, which are not yet well understood.
Holstein model and quantum bipolarons
The Holstein model is a theoretical framework used to describe the interaction between electrons and phonons. It has previously been used to study the generic physics of linear electron-phonon coupling.
The researchers extended the Holstein model to include quadratic electron-phonon coupling in their study.
The Holstein model helps calculate quantities such as the binding energy of Cooper pairs and the critical temperature of superconductors.
In conventional materials, the binding of electrons, mediated by phonons, leads to the formation of Cooper pairs.
The interaction is linear, meaning that the strength of the coupling increases with the amplitude of lattice vibrations. This interaction can be understood using classical physical principles and is well supported by experimental observations such as isotope effects.
This is completely different with quadratic coupling. By extending the Holstein model to include the second-order dependence of the coupling on the displacement of phonons, the researchers take into account quantum fluctuations (random motion) of phonons and zero-point energy (the energy of phonons at 0 Kelvin).
The electrons interact with the quantum fluctuations of phonons to form ‘quantum bipolarons’. Unlike linear coupling, the origin of the attractive interactions is purely quantum mechanical.
Superconductivity in weak and strong coupling limit
The researchers found that when the electron-phonon interaction is weak, the mechanism by which electrons pair up to form Cooper pairs is ineffective, similar to the linear case. This leads to a low critical temperature that can be influenced by the mass of the ions (isotope effect), but in a different way than in the linear case.
In other words: the (low) critical temperature of the material can change significantly with different atomic masses.
In contrast, when electron-phonon interactions are strong, this results in the formation of quantum bipolarons, which can become superconducting at a temperature determined by their effective mass and density.
Below the critical temperature, the condensate of quantum bipolarons can move freely without disturbing the crystal. More mobility leads to a superconducting state, which is more stable and has a higher critical temperature. In contrast to the linear mechanism, the quantum bipolar mass is only slightly increased by coupling, allowing higher critical temperatures.
“Our work shows that this mechanism allows higher transition temperatures, at least for strong coupling. What is also good is that this mechanism does not require any special boundary conditions to function, and there are quite realistic conditions in which it will be dominant,” he explains. Dr. Volkov.
Han predicted: “Based on fundamental physical constants relevant to solid materials, an optimistic estimate of the critical temperature achievable by this mechanism may be on the order of 100 Kelvin.”
Future work
“The possible implication would primarily be an improvement in the superconducting transition temperature. Superconductivity also depends sensitively on the properties of electrons; to achieve strong coupling we therefore propose to use specially designed superlattices for electrons,” explains Dr. Volkov out.
The researchers mention that theoretically the next step would be to find the optimal coupling strength regime for superconductivity. The researchers also hope that experimentalists will investigate superlattice materials with large quadratic electron-phonon couplings.
“Experimentally, creating superlattices via patterning or using interfaces between twisted materials could be a promising avenue to realize the type of superconductivity we predicted,” said Dr. Volkov.
Han also pointed out, “It is critical to identify materials with large quadratic electron-phonon couplings based on ab initio calculations, as this has not been systematically investigated.”
More information:
Zhaoyu Han et al., Quantum Bipolaron Superconductivity by Quadratic Electron-Phonon Coupling, Physical Assessment Letters (2024). DOI: 10.1103/PhysRevLett.132.226001. On arXiv: DOI: 10.48550/arxiv.2312.03844
© 2024 Science X Network
Quote: High-temperature superconductivity: exploring quadratic electron-phonon coupling (2024, June 20) retrieved June 21, 2024 from https://phys.org/news/2024-06-high-temperature-superconductivity-exploring-quadratic.html
This document is copyrighted. Except for fair dealing purposes for the purpose of private study or research, no part may be reproduced without written permission. The content is provided for informational purposes only.